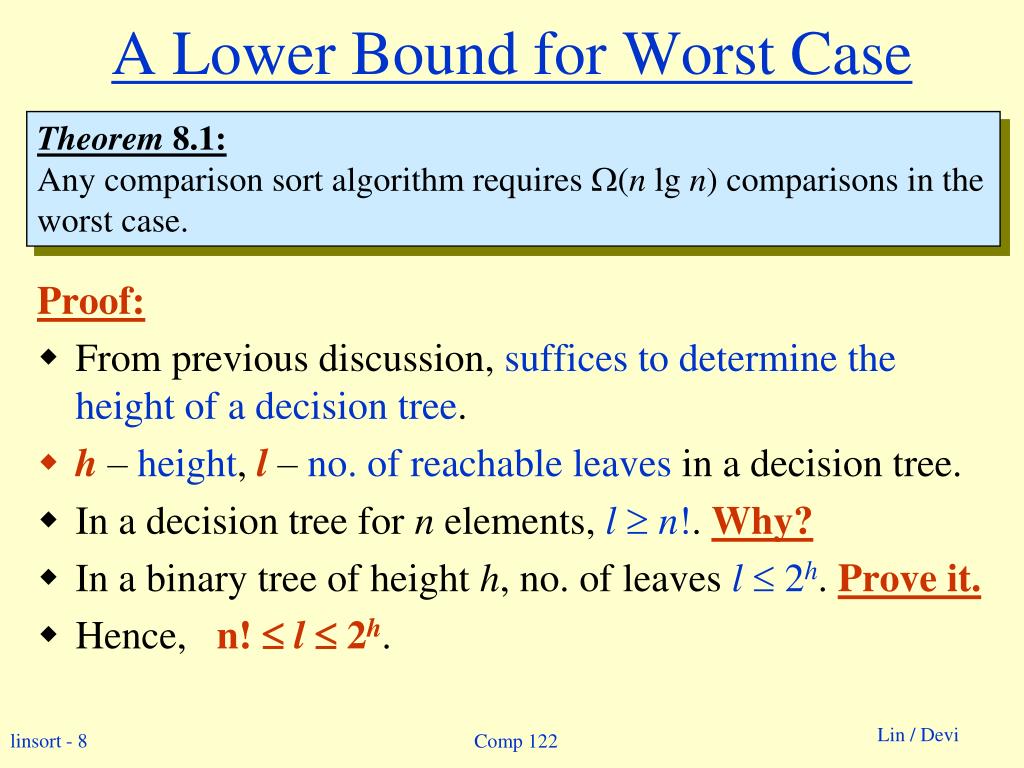
Worst Case Lower Bound . According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. worst case lower bound can be said to be ω(n^2). The best case running time can be said to be θ(n), when the input is. lower bound theory: Also, every algorithm must take at least l(n) time in the worst case. a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n.
from www.slideserve.com
worst case lower bound can be said to be ω(n^2). asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. The best case running time can be said to be θ(n), when the input is. a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n. Also, every algorithm must take at least l(n) time in the worst case. lower bound theory: According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input.
PPT Lower Bounds & Sorting in Linear Time PowerPoint Presentation
Worst Case Lower Bound worst case lower bound can be said to be ω(n^2). According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. Also, every algorithm must take at least l(n) time in the worst case. worst case lower bound can be said to be ω(n^2). a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n. The best case running time can be said to be θ(n), when the input is. asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. lower bound theory:
From www.youtube.com
Lower bounds on worst case of comparison sorting Linear Time Sorting Worst Case Lower Bound According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. lower bound theory: The best case running time can be said to be θ(n), when the input is. asignificantexampleofalowerbounds argument is. Worst Case Lower Bound.
From www.researchgate.net
Convergence characteristics. Upper bounds for all cases and lower bound Worst Case Lower Bound The best case running time can be said to be θ(n), when the input is. worst case lower bound can be said to be ω(n^2). Also, every algorithm must take at least l(n) time in the worst case. lower bound theory: asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in. Worst Case Lower Bound.
From www.researchgate.net
(PDF) A Tight Lower Bound for the Worst Case of BottomUp Heapsort. Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. The best case running time can be said to be θ(n), when the input is. a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n. asignificantexampleofalowerbounds argument is the proof from section 7.9. Worst Case Lower Bound.
From www.slideserve.com
PPT Algorithms and Computations Complexity Lecture 3 Growth of Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. worst case lower bound can be said to be ω(n^2). The best case running time can be said to be θ(n), when the input is. According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any. Worst Case Lower Bound.
From suwieramtow.blogspot.com
Upper And Lower Bounds Upper and Lower Bounds YouTube The Worst Case Lower Bound a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n. lower bound theory: asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. worst case lower bound can be said to be ω(n^2). The best case running. Worst Case Lower Bound.
From www.slideserve.com
PPT The Lower Bounds of Problems PowerPoint Presentation, free Worst Case Lower Bound lower bound theory: According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. Also, every algorithm must take at least l(n) time in the worst case. a lower bound for some. Worst Case Lower Bound.
From www.researchgate.net
Wind/load upper/lower bound and its worstcase scenario with Worst Case Lower Bound According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. worst case lower bound can be said to be ω(n^2). Also, every algorithm must take at least l(n) time in the worst. Worst Case Lower Bound.
From www.researchgate.net
WORST CASE BOUNDS FOR THE CONSIDERED METRICS Download Table Worst Case Lower Bound lower bound theory: According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. a lower bound for some problem and some length n, is obtained by the negation of an upper. Worst Case Lower Bound.
From www.researchgate.net
Worst Case Bounds for States in Which Taxes Increased. Download Worst Case Lower Bound According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. lower bound theory: The best case running time can be said to be θ(n), when the input is. Also, every algorithm must. Worst Case Lower Bound.
From www.researchgate.net
(PDF) Lower Bounds on the WorstCase Complexity of Efficient Global Worst Case Lower Bound According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. The best case running time can be said to be θ(n), when the input is. lower bound theory: asignificantexampleofalowerbounds argument is. Worst Case Lower Bound.
From www.slideserve.com
PPT Algorithms and Computations Complexity Lecture 3 Growth of Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. The best case running time can be said to be θ(n), when the input is. lower bound theory: According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose. Worst Case Lower Bound.
From www.researchgate.net
1 The computed worstcase bounds on the HBM and FGM versus the Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time complexity is less than l(n) for random input. asignificantexampleofalowerbounds argument is the proof from section 7.9 that. Worst Case Lower Bound.
From subscription.packtpub.com
Lower bounds for sorting R Data Structures and Algorithms Worst Case Lower Bound a lower bound for some problem and some length n, is obtained by the negation of an upper bound for that n. worst case lower bound can be said to be ω(n^2). According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common. Worst Case Lower Bound.
From deepai.org
Improved WorstCase Regret Bounds for Randomized LeastSquares Value Worst Case Lower Bound lower bound theory: asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. worst case lower bound can be said to be ω(n^2). According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem). Worst Case Lower Bound.
From www.slideserve.com
PPT Lower Bounds & Sorting in Linear Time PowerPoint Presentation Worst Case Lower Bound The best case running time can be said to be θ(n), when the input is. Also, every algorithm must take at least l(n) time in the worst case. asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. lower bound theory: worst case lower bound can be said to be. Worst Case Lower Bound.
From www.slideserve.com
PPT Sampling Lower Bounds via Information Theory PowerPoint Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not possible to have any other algorithm (for a common problem) whose time. Worst Case Lower Bound.
From www.researchgate.net
Comparison of the worstcase and datadependent bounds on the gradient Worst Case Lower Bound Also, every algorithm must take at least l(n) time in the worst case. asignificantexampleofalowerbounds argument is the proof from section 7.9 that the problem of sorting is o(nlogn) in the. The best case running time can be said to be θ(n), when the input is. lower bound theory: a lower bound for some problem and some length. Worst Case Lower Bound.
From www.researchgate.net
Zone 3 protection on line 2324, worstcase bounds for 1.8 ≤ ηp, ηq ≤ Worst Case Lower Bound lower bound theory: worst case lower bound can be said to be ω(n^2). Also, every algorithm must take at least l(n) time in the worst case. The best case running time can be said to be θ(n), when the input is. According to the lower bound theory, for a lower bound l(n) of an algorithm, it is not. Worst Case Lower Bound.